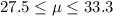Answer:
The 95% CI is
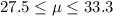
The margin of error is 2.9 minutes.
Explanation:
The question is incomplete:
The sample standard deviation was 7.3 minutes.
Assume the population is normally distributed and use a t-distribution to construct a 95% confidence interval for the population mean mu.
The sample mean is 30.4
The sample standard deviation is 7.3
The sample size is 27.
The degrees of freedom are:
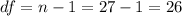
For a 95% CI and df=26, the critical value for t is t=2.056.
The margin of error can be calculated as:
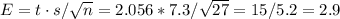
The lower and upper bounds of the 95% CI are:
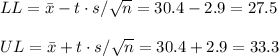
The 95% CI is then