Answer:
The correct answer is because the alternative terms are prime and composite.
Explanation:
The given polynomial is
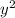 + 7.
+ 7.
The value of the polynomial when y = 0 is 7.
The value of the polynomial when y = 1 is 8.
The value of the polynomial when y = 2 is 11.
The value of the polynomial when y = 3 is 16.
The value of the polynomial when y = 4 is 23.
The value of the polynomial when y = 5 is 32.
The value of the polynomial when y = 6 is 43.
And so on. Thus the value of the polynomial at alternate values of y are prime. Thus this is why the polynomial is said to be relatively prime.