Answer:
The speed is 2.427 x10⁸ m/s
Step-by-step explanation:
The equation for measured time is the following:
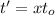
Where
x = 0.587
Replacing:
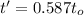
The equation for the speed is:
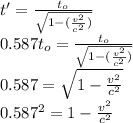
Rearrange the expression:
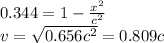
Where c = 3x10⁸m/s
Replacing:
v = 0.809 * 3x10⁸ = 2.427 x10⁸ m/s