Answer:
2.04 m
Step-by-step explanation:
Parameters given:
Mass of box, m = 10 kg
Initial speed of box, u = 2 m/s
Final velocity of box, v = 0 m/s (The box comes to rest)
Coefficient of friction, μ = 0.1
To find the distance moved by the box, we need to use one of Newton's equation of linear motion:
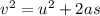
where a = acceleration and s = distance moved
We don't have acceleration, a, but we can get this by first finding the frictional force. This is because we know that the frictional force will have equal magnitude but opposite direction as the force exerted by the box on the floor.
Frictional force is given as:
Fr = μmg
where g = acceleration due to gravity
Fr = 0.1 * 10 * 9.8
Fr = 9.8 N
The force exerted by the box on the floor will be -9.8 N.
Force is given as:
F = ma
-9.8 = 10 * a
a = -9.8/10 = -0.98
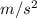
This shows that it is a deceleration because the box is slowing down.
Going back to the equation of motion:
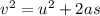
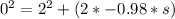
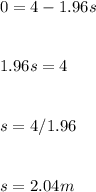
The distance moved by the box is 2.04 m