Answer:
(a) The acceleration is 0.608
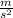
(b) The minimum value of coefficient of static friction is 0.062
Step-by-step explanation:
Given:
Angular frequency
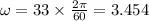
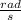
Distance between axis of rotation and watermelon
 m
m
(a)
For calculating acceleration,
From the formula of centripetal acceleration,
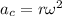
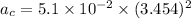
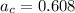
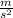
(b)
For finding the minimum value of the coefficient of static friction,
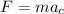
But here only friction force act
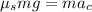
Where
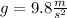
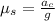
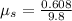
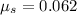
Therefore, the acceleration is 0.608
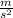 and minimum value of coefficient of static friction is 0.062
and minimum value of coefficient of static friction is 0.062