Question

Source 1 can supply energy at the rate of 11000 kJ/min at 310°C. A second Source 2 can supply energy at the rate of 110000 kJ/min at 65°C. Which source (1 or 2) would you choose to supply energy to an ideal reversible heat engine that is to produce the larger amount of power if the temperature of the surroundings is 38°C?

Answer 1

Source 2.

Step-by-step explanation:

The efficiency of the ideal reversible heat engine is given by the Carnot's power cycle:

`\eta_(th) = 1 - (T_(L))/(T_(H))`

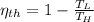
Where:

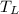`T_(L)` - Temperature of the cold reservoir, in K.

`T_(H)` - Temperature of the hot reservoir, in K.

The thermal efficiencies are, respectively:

Source 1

`\eta_(th) = 1 - (311.15\,K)/(583.15\,K)`

`\eta_(th) = 0.466 \,(46.6\,\%)`

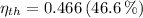
Source 2

`\eta_(th) = 1 - (311.15\,K)/(338.15\,K)`

`\eta_(th) = 0.0798 \,(7.98\,\%)`

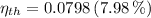
The power produced by each device is presented below:

Source 1

`\dot W = (0.466)\cdot (11000\,(kJ)/(min))\cdot ((1\,min)/(60\,s) )`

`\dot W = 85.433\,kW`

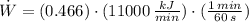
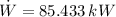
Source 2

`\dot W = (0.0798)\cdot (110000\,(kJ)/(min))\cdot ((1\,min)/(60\,s) )`

`\dot W = 146.3\,kW`

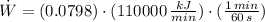
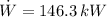
The source 2 produces the largest amount of power.