Answer:
0.39
Step-by-step explanation:
Parameters given:
Mass of car, m = 940 kg
Speed of car, v = 11.8 m/s
Power supplied by engine, P = 4300 W
To get the acceleration, we must define the relationship between Power and velocity.
Power, P, is given in terms of velocity, v, as:
P = F * v
where F = force
This is because Power is given as:
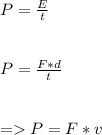
(where E = energy. t = time taken, d = distance moved)
Force, F, is given as:
F = m*a
Therefore, Power will be:
P = m * a * v
Acceleration, a, will then be:
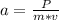
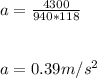
The acceleration of the car at that time is 0.39