Question

A line passing through the points (6, –2) and (–2, 4).

Complete the work shown:

1. Use slope formula to find the slope.

2. Substitute a point and slope in point-slope form.

3. Distribute the slope through the parentheses.

4. Solve for the y-variable.

1. m = StartFraction 4 minus (negative 2) Over negative 2 minus 6 EndFraction = StartFraction 6 Over negative 8 EndFraction = negative three-fourths. 2. y minus 4 = negative three-fourths (x minus (negative 2)). 3. y minus 4 = negative three-fourths x minus three-halves.

4. y = negative three-fourths x +

Answer 1

its 2.5

Explanation:

Answer 2

`y=(-3)/(4)x+(5)/(2)`

Explanation:

Our given points: (6, –2) and (–2, 4).

1. Use the slope formula to find the slope

`m=(y_(2) -y_(1))/(x_(2)-x_(1))`

`m=(-2-4)/(6-(-2))`

`m=(-6)/(8)`

`m=(-3)/(4)`

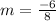
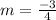
Therefore, the slope of the line is
`(-3)/(4)`.

2. Substitute a point and a slope in point-slope form

Point-slope form:
`y_(2)-y_(1)=m(x_(2)-x_(1))`

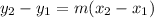
In point-slope form, the variables
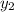`y_(2)` and
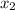`x_(2)` stay y and x, so when we plug a point into this equation, we plug the x and y variables in
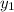`y_(1)` and
`x_(1)`. We can plug in either given point, (6, –2) and (–2, 4), for this equation to be correct. Below, you can see that I plugged in the first given point.

`y_(2)-y_(1)=m(x_(2)-x_(1))\\y-(-2)=m(x-6)\\y+2=m(x-6)\\y+2=(-3)/(4)(x-6)`

3. Distribute the slope through the parentheses

`y+2=(-3)/(4)(x-6)\\y+2=(-3)/(4)x-((-3)/(4))(6)\\y+2=(-3)/(4)x-(-9)/(2)\\y+2=(-3)/(2)+(9)/(2)`

4. Solve for the y-variable

This is asking us to isolate the y variable in the equation that we have created in step 3. To isolate the y-variable, all we have to do is move the +2 over to the right side by subtracting both sides by 2.

`y+2=(-3)/(4)x+(9)/(2)`

`y=(-3)/(4)x+(9)/(2)-2`

`y=(-3)/(4)x+(9)/(2)-(4)/(2)`

`y=(-3)/(4)x+(5)/(2)`

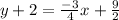
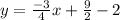
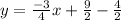
I hope this helps!