Question

The fraction of defective integrated circuits produced in a photolithography process is being studied. A random sample of 300 circuits is tested, revealing 16 defectives. Part 1 (a) Use the data to test the hypothesis that the proportion is not 0.04. Use α =0.05. Round the answer to 2 decimal places.

Answer 1

The test statistic

Z = 1.149

Since the calculated value of Z = 1.149 is less than 1.96 at 5% (0.05) level of significance.

The null hypothesis is accepted

Hence the proportion is not equal 0.04

Explanation:

Given data a random sample of 300 circuits is tested, revealing 16 defectives.

The proportion of success

`p = (16)/(300) =0.053`

Null hypothesis:- H₀ = P ≠0.04

Alternative hypothesis:- H₁ = P =0.04

Q = 1-P = 1-0.04=0.96

Level of significance ∝ =0.05

The test statistic

`Z= \frac{p-P}{\sqrt{(PQ)/(n) } }`

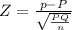
now substitute all values, we get

`Z= \frac{0.053-0.04}{\sqrt{(0.0384)/(300) } }`

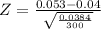
on calculation, Z = 1.149

Since the calculated value of Z = 1.149 is less than 1.96 at 5% (0.05) level of significance.

The null hypothesis is accepted .

Hence the proportion is not equal 0.04