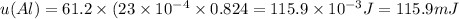Question

A solenoid that is 82.4 cm long has a cross-sectional area of 23.0 cm2. There are 1380 turns of wire carrying a current of 5.89 A. (a) Calculate the energy density of the magnetic field inside the solenoid. (b) Find the total energy in joules stored in the magnetic field there (neglect end effects).

Answer 1

Answer with Explanation:

We are given that

Length of solenoid,l=82.4 cm=
`(82.4)/(100)=0.824 m`

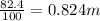
1m=100 cm

Area,A=
`23.0 cm^2=23* 10^(-4) m^2`

`1cm^2=10^(-4) m^2`

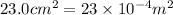
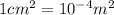
Number of turns,N=1380

Current,I=5.89 A

a.Magnetic field of solenoid,
`B=(\mu_0 NI)/(l)`

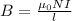
Where
`\mu_0=4\pi* 10^(-7)`

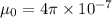
Using the formula

`B=(4\pi* 10^(-7)* 5.89* 1380)/(0.824)=0.0124 T`

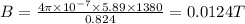
Energy density of the magnetic field inside the solenoid=
`u=(B^2)/(2\mu_0)=((0.0124)^2)/(2* 4\pi* 10^(-7))=61.2 J/m^3`

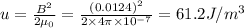
b.Total energy stored in the magnetic field=
`u(Al)=61.2* (23* 10^(-4)* 0.824=115.9* 10^(-3) J=115.9 mJ`

`1mJ=10^(-3) J`