Answer:
The coordinates of the target are (8790m, 3400m).
Step-by-step explanation:
First of all, we have to find the components of the initial velocity
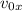 and
and
 , using trigonometry:
, using trigonometry:

Now, we find the x-coordinate using the equation of motion with constant speed (since there is no external force in x-axis that causes an horizontal acceleration):
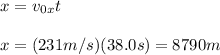
Then, we find the y-coordinate using the equation of position of an object with constant acceleration (since there is the gravitational force causing a vertical acceleration on the shell):
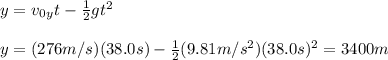
Finally, the coordinates of the target are (8790m, 3400m).