Question

Assume a test for cancer correctly identifies 98% of the people tested who do have cancer. Unfortunately, the test gives a false positive reading 1.5% of the time. (A false positive is when the test says you have cancer, but you actually do not.) Millions of people are tested and 0.6% actually have cancer. Find the probability that a person with a positive test result has cancer

Answer 1

0.2828.

Explanation:

From the information given:

• =98%=0.98
• P(Positive|No Cancer)=1.5%=0.015
• P(Cancer)=0.6%=0.006

Therefore: P(No Cancer)=1-P(Cancer)=1-0.006=0.994

We want to determine the probability that a person with a positive test result has cancer. i,e. P(Cancer|Positive)

Using Bayes Theorem for Conditional Probability

`P(Cancer|Positive)=(P(Positive|Cancer)P(Cancer))/(P(Positive|Cancer)P(Cancer)+P(Positive|No Cancer)P(No Cancer))\\=(0.98X0.006)/(0.98X0.006+0.015X0.994)`
`P(Cancer|Positive)=0.2828`

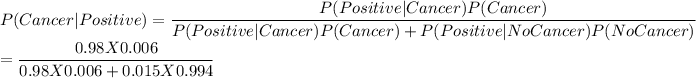
Therefore, the probability that a person with a positive test result has cancer is 0.2828.