Question

For a solid-state transformation that obeys the Avrami equation, the parameter n has been determined to have a value of 1.5. The reaction takes 125 seconds to reach 30% completion. What is the total time (in seconds) for the transformation to reach 95% completion

Answer 1

The total time (in seconds) for the transformation to reach 95% completion is 516.783 secs

Step-by-step explanation:

Given Data:

n=1.5

Time to reach 30% completion=125 Secs

Required:

Total Time for the transformation to reach 95% completion=?

Solution:

Avrami Equation:

`y=1-e^(-kt^n)`

where:

k is time constant

t is the time

y is the remaining amount of reaction.

First Calculate K:

`0.3-1=-e^(-k*125^1.5)\\-0.7=-e^(-k*125^1.5)\\`

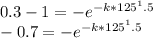
Taking "ln"on both sides

ln(0.7)=-k*(125^1.5)

k=0.000255

At 95 % completion:

Avrami Equation Can be rearranged as:

`y-1=-e^(-kt^n)\\ln(-y+1)=-k*t^n\\(ln(-y+1))/(-k)=t^n\\ ln((ln(-y+1))/(-k))=n*lnt\\(ln((ln(-y+1))/(-k)))/(n)=lnt \\e^{(ln((ln(-y+1))/(-k)))/(n)}=t`

`e^{(ln((ln(-0.95+1))/(-0.000255))))/(1.5)}=t\\t=516.783 \ secs`

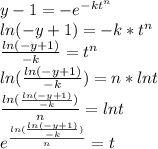
The total time (in seconds) for the transformation to reach 95% completion is 516.783 secs