Question

A population of values his normal distribution with men equal to 27.6 and standard deviation equal 39.4 do you intend to draw a random sample of size N equal 173 what is the mean of the distribution of sample means

Answer 1

The mean of the distribution of sample means is 27.6

Explanation:

We are given the following in the question:

Mean, μ = 27.6

Standard Deviation, σ = 39.4

We are given that the population is a bell shaped distribution that is a normal distribution.

Sample size, n = 173.

We have to find the mean of the distribution of sample means.

Central Limit theorem:

• It states that the distribution of the sample means approximate the normal distribution as the sample size increases.
• The mean of all samples from the same population will be approximately equal to the mean of the population.

Thus, we can write:

`\mu_{\bar{x}} = \mu = 27.6`

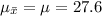
Thus, the mean of the distribution of sample means is 27.6