Answer:
The impulse on the ball delivered by the floor is 2.52 kg-m/s.
Step-by-step explanation:
Given that,
Mass of the ball, m = 0.22 kg
It is dropped from an initial height of 1.80 m. It rebounds back after colliding with the floor to a final height of 1.50 m. Initial velocity and final velocity can be calculated using conservation of energy as :

Final velocity,
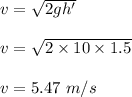
As the ball rebounds, v = -5.47 m/s
We need to find the impulse on the ball delivered by the floor. We know that impulse is equal to the change in momentum as follows :

So, the impulse on the ball delivered by the floor is 2.52 kg-m/s.