Question

The U.S. mint reports that some of the coins in circulation are in fact not fair, but others are fair. You flip a coin 100 times and it lands on heads 65 times. (a) Perform a hypothesis test by doing the following steps • State the null and alternative hypotheses. Specify if it is a one-sided or two-sided test. • Find the z-statistic AND the p-value. • Decide whether to reject or fail to reject the null hypothesis based on a significance level of α = .05. (b) The mint specifies that 99.9% of all coins are fair, and .1% of coins are weighted. The weighted coins all have a 65% probability of landing on heads. Use Bayes’ Theorem to estimate the probability that the coin is weighted. (Note: If your calculator cannot compute the probabilities involved, use the dbinom() function in R to calculate them.) For example if you wanted to find the probability that a binomial random variable with n = 100 and p = .59 would have 60 successes you could enter the following into R: > dbinom(x = 60, size = 100, prob = .59) [1] 0.07955339 (c) Compare your conclusions under the hypothesis test and using Bayes’ theorem. Are they similar or different? Why do the two approaches give you similar/different conclusions?

Answer 1

We are given:

n = 100

x(number of heads) = 65

Let's take P= propoortion of head

a) •for null hypothesis:

`H_0 : P = 0.5`

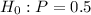
For alternative hypothesis:

`H_1 : P ≠ 0.5`

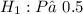
Since it's a 2 tailed test, we have:

`p' = (x)/(n) = (65)/(100) = 0.65`

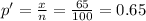
For z-statistic:

`Z = \frac{p'-0.5}{\sqrt{(0.5(1-0.5))/(n)}} =`

`= \frac{0.65-0.5}{\sqrt{(0.5(1-0.5))/(100)}}`

`=(0.15)/(0.05) = 3`

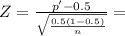
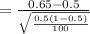
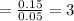
For P value:

2P(z>3) = 2*0.0013

= 0.0026

•As P value (0.0026) is less than level of significance (0.5), we reject the null hypothesis.

b) we are given:

P( fair coins) = 0.999

P(coins not fair) = 0.001

P(head| fair coins) = 0.5

P(head| coins not fair) = 0.65

P(coin weighted|head) will be:

`P(W|H) = (P(W.coin)•P(H.coin))/(P(W.coin)•P(H|W.coin)+P(F.coin)*P(H|F.coin))`

`= (0.001*0.65)/(0.001*0.65+0.999*0.5)`

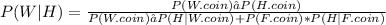
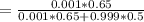
= 0.0013

c) Comparing the conclusions of part (a) and (b) under the hypothesis test we see that they yielded different results.

Using Bayes' theorem, we can see that the probability of the coin being unfair is very unlikely (less) but the null hypothesis tells us otherwise.

The different conclusions gotten are due to methods used or different confidence level.