Answer:
The correct answer is by allotting rooms of nth guest of nth bus to nth power of nth prime numbers.
Explanation:
There are infinite number of rooms in Hilbert's Grand Hotel.
The hotel is empty. Now countably infinitely many buses show up.
Bus 1 has 1 guest, Bus 2 has 2 guests, Bus 3 has 3 guests, and so on.
Thus the hotel accommodates guest of bus 1 to room number 2, the first prime number.
Again the hotel accommodates 2 guests of bus 2 to room number 3 (the next prime number) and
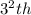 room.
room.
Again the hotel accommodates 3 guests of bus 3 to room number 5(the next prime number);
 room; and
room; and
 room.
room.
As there are infinitely many prime numbers, this allocation is feasible and there would be no overlapping rooms.
This way the hotel can accommodate countably infinitely many buses with each guests having individual rooms.