Answer:
The firm save $385.308 annually in ordering and carrying costs by using the EOQ
Step-by-step explanation:
Given:
- Demand in a month (u) : 786 => the demand in a year (D): 786*12 = 9432
- The holding cost :35 percent of the purchase price per crate (C) = 0.35*9 =$3.15
- Ordering costs (O) : $27
To find the money the company can save when ordering based on the EOQ, we use the following formula to find the EOQ:
EOQ =
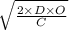 =
=
 $402.1
$402.1
- The total cost using the EOQ
= [(EOQ / 2) x C] + [(D / EOQ) x 0]
= (402.1 /2)*3.15 + (9432 / 402.1) x 27
= $1266.642
- The actual total annual inventory cost:
TC = [(u / 2) x C] + [(D / u) x O]
= [(786 / 2) x 3.15] + [(9432 / 786) x 27]
= 1327.95 + 324
= $1651.95
- The difference between the actual total cost minus the EOQ cost is:
= $1651.95 - $1266.642
=$385.308
So the firm save $385.308 annually in ordering and carrying costs by using the EOQ