Answer:
The work done required on the coin during the displacement is 21.75 w.
Step-by-step explanation:
Given that,
A coin slides over a friction-less plane i.e friction force = 0.
The co-ordinate of the given point is (1.40 m, 7.20 m).
The position vector of the given point is represented by
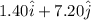 .
.
The displacement of the coin is
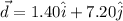
The force has magnitude 4.50 N and its makes an angle 128° with positive x axis.
Then x component of the force = 4.50 cos128°
The y component of the force = 4.50 sin128°
Then the position vector of the force is
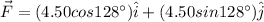
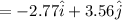
We know that,
work done is a scalar product of force and displacement.
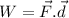

=(-2.77×1.40+ 3.56×7.20) w
=21.75 w
The work done required on the coin during the displacement is 21.75 w.