Given:
The exterior angle P is 74°
The measure of ∠PRQ is 51°
We need to determine the measure of ∠PQR
Measure of ∠QPR:
From the figure, it is obvious that P is the intersection of the two lines.
The angle 74° and ∠QPR are vertically opposite angles.
Since, vertically opposite angles are always equal, then the measure of ∠QPR is 74°
Thus, the measure of ∠QPR is 74°
Measure of ∠PQR:
The measure of ∠PQR can be determined using the triangle sum property.
Thus, we have;
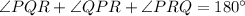
Substituting the values, we get;
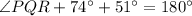
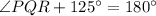
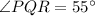
Thus, the measure of ∠PQR is 55°
Hence, Option B is the correct answer.