Question

Solve the equation: 2x^2(x–1)(x–3)(x+2)(x–7)(x+3)(x–9)(x+7)(2x–15)(3x+18)=0

Answer 1

I assuming by "solve the equation" you mean I have to find the zeroes of this equation
(i.e the values of x which satisfy this equation). For that any one of the factor value must be equal to 0 so that when it will multiply with other factors it will make the left hand side equal to right hand side giving us one of the possible solutions. Since there are 10 different factors there will be 10 different solutions here to x.

they are:
(in order of the factors mentioned in the question)

(i)

`2 {x}^(2) = 0 \\ x = 0`

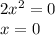
(ii)

`x - 1 = 0 \\ x = 1`

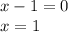
(iii)

`x - 3 = 0 \\ x = 3`

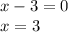
(iv)

x+2=0
x=-2

(v)
x-7=0
x= 7

(vi)

x+3=0
x=-3

(vii)

x-9=0
x=9

(viii)
x+7=0
x=-7

(ix)

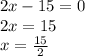
`2x - 15 = 0 \\ 2x = 15 \\ x = (15)/(2)`

(x)

`3x + 18 = 0 \\ 3x = - 18 \\ x = - 6`