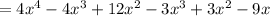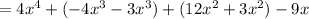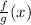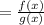Answer:
(f+g)(x)=5x²-4x+3
(f-g)(x)=3x²-2x+3
(fg)(x)

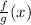

Explanation:
Given that,
f(x)=4x²-3x
g(x)=x²-x+3
(f+g)(x)
=f(x)+g(x)
=4x²-3x+x²-x+3
=(4x²+x²)+(-3x-x)+3 [ combined the like terms]
=5x²-4x+3
(f-g)(x)
=f(x)-g(x)
=4x²-3x-(x²-x+3)
=4x²-3x-x²+x-3
=(4x²-x²)+(-3x+x)-3 [ combined the like terms]
=3x²-2x+3
(fg)(x)
=f(x).g(x)
=(4x²-3x).(x²-x+3)
=4x²(x²-x+3)-3x(x²-x+3)