Given:
A ball is kicked 4 feet above the ground with an initial vertical velocity of 55 feet per second.
The function
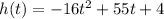 represents the height h(in feet) of the ball after t seconds.
represents the height h(in feet) of the ball after t seconds.
We need to determine the time of the ball at which it is 30 feet above the ground.
Time:
To determine the time that it takes for the ball to reach a height of 30 feet above the ground, let us substitute h(t) = 30, we get;
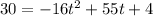
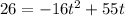
Adding both sides of the equation by 16t², we get;
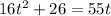
Subtracting both sides of the equation by 55t, we have;

Let us solve the quadratic equation using the quadratic formula, we get;


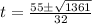
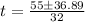
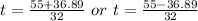


The value of t is t = 0.6 because this denotes the time taken by the ball to reach a height of 30 feet from the ground.
Therefore, the time taken by the ball to reach a height of 30 feet above the ground is 0.6 seconds.