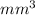Question

A pharmaticeutical company is making a new medicine capsule shaped like a cylinder with a half sphere at each end. The

cylinder portion of the capsule is 8 millimeters long and has a diameter of 6 millimeters.
What is the volume of the figure? Use 3.14 for
Round to the nearest hundredth.

Answer 1

339.12 cubic millimeters

Explanation:

I just took test on edge2020

Answer 2

The volume of the capsule is: 339.12
`mm^3`

Explanation:

Notice that the total capsule is made by adding the volume of the cylinder central part, plus the volume of two half spheres at the ends (which makes for the addition of the volume of a full sphere of the same radius as the cylinder).

So let's first calculate the volume of the cylinder that has diameter 6 mm (so a radius of 3 mm), and height 8 mm:

Volume of the cylinder = area of the base (a circle of radius 3 mm in our case) times the height (8 mm in our case) :

`V_c=\pi \,r^2\,*\,h\\V_c=\pi \,(3\,mm)^2\,(8\,mm)\\V_c=226.08\,mm^3`

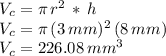
Now for the volume of a full sphere of radius 3 mm, we use the formula for the sphere's volume:

`V_s=(4)/(3) \,\pi \,r^3\\V_s=(4)/(3) \,\pi \,(3\,mm)^3\\\\V_s=113.04\,mm^3`

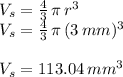
Therefore, the total volume of the capsule is the addition of these two volumes:

Volume of the capsule = (226.08 + 113.04)
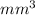`mm^3` 339.12
`mm^3`