Answer:
Decayed mass: 909.56 g, Left mass: 14.44 g.
Step-by-step explanation:
The time constant of the Chromium-51 is:
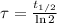
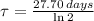
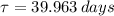
The decay equation for the isotope has the following form:
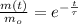
The total decay at a given instant t is:

The total decay at 166.2 days is:
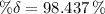
Decayed mass: 909.56 g, Left mass: 14.44 g.