Answer:
585 nm
Step-by-step explanation:
The formula that gives the position of the m-th maximum (bright fringe) relative to the central maximum in the interference pattern produced by diffraction from double slit is:


where
m is the order of the maximum
 is the wavelength
is the wavelength
D is the distance of the screen from the slits
d is the separation between the slits
The distance between two consecutive bright fringes therefore is given by:
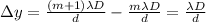
In this problem we have:
 (distance between two bright fringes)
(distance between two bright fringes)
D = 2.0 m (distance of the screen)
d = 3.0 x 10−3 m (separation between the slits)
Solving for
 , we find the wavelength:
, we find the wavelength:
