Answer:
We get the value of
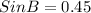 in radians which is equal to
in radians which is equal to
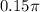
Explanation:
Given that,
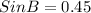
To find:- find angle B in Radians.
So,
To find the Angle B needs to take
 . Sin has a range
. Sin has a range
![[-1,1]](https://img.qammunity.org/2021/formulas/mathematics/high-school/ic03iz4me3a0qwlx3l0inzcj87uwq6m1v4.png) for all
for all
 ∈
∈
 .
.
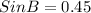
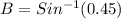

Thus angle
 is in degree.
is in degree.
Here, converting the degree into radian we find,
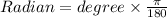
⇒
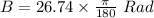
⇒

Therefore,
We get the value of
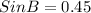 in radians which is equal to
in radians which is equal to
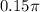 .
.