Answer:
The height is increasing at the rate of 0.42 ft/min
Explanation:
Given that:
The diameter = height
and
diameter d = 2 r
2 r = h
r =
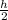
The volume of the cone formed = v
v ⇒
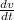 = 40 ft³/ min
= 40 ft³/ min
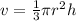



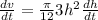
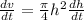
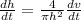
at h = 11 ft high

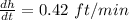
Hence, the height of the pile was increasing at the rate of 0.42 ft/min