Question

I start with $9-2x$ widgets. Each day, I produce $2x+1$ widgets, and destroy $3-x$ widgets. After three days, how many widgets am I left with, in terms of $x$?

Answer 1

Each day, the net gain/loss in widgets is (2x+1)-(3-x) = 3x-2 widgets. In three days, this difference becomes 3 * (3x-2) = 9x-6. Adding this to our initial amount of widgets, we have (9-2x)+(9x-6). Combining like terms, we have (9x-2x)+(9-6) = 7x+3, or 3+7x.

Answer 2

3+7x

Explanation:

-We add the number of widgets produced in 3 days to the number originally owned:

`T_(own)=(9-2x)+3(2x+1)\\\\=9-2x+6x+3\\\\=12+4x`

#We the determine the widgets destroyed in 3 days:

`T_(destroy)=3(3-x)\\\\=9-3x`

#Subtract the widgets destroyed from the total owned to get what is left after 3 days:

`Remain=T_(own)-T_(destroy)\\\\=(12+4x)-(9-3x)\\\\=12+4x-9+3x\\\\=3+7x`

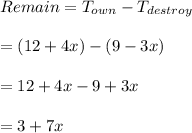
Hence, the number of widgets remaining after 3 days is 3+7x