Answer:
Its speed is
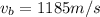 .
.
Step-by-step explanation:
The angular momentum is defined as:
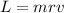 (1)
(1)
Since there is no torque acting on the system, it can be express in the following way:
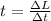
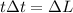

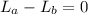
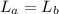 (2)
(2)
Replacing equation 1 in equation 2 it is gotten:
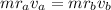 (3)
(3)
As can be seen in equation 3 the angular momentum is conserved.
Where m is the mass of the comet,
 is the orbital radius when it is farther form the Sun,
is the orbital radius when it is farther form the Sun,
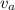 is the speed when is farther from the Sun,
is the speed when is farther from the Sun,
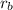 is the orbital radius when is closer to the Sun,
is the orbital radius when is closer to the Sun,
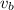 is the speed when it is closer to the Sun.
is the speed when it is closer to the Sun.
From equation 3
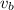 will be isolated:
will be isolated:
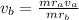
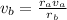 (4)
(4)
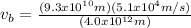
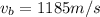
Hence, its speed is
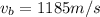 .
.