Question

The body mass index, BMI=(W(kg))/(H^2 (m^2)) of a 1.7-m-tall woman who normally has pasta for lunch is 30. She now decides to drop the oil that she uses to prepare her lunch (2 tablespoons, corresponding to 240 calories). Assuming that the deficit in the calorie intake is made up by burning body fat, determine how long it will take for the BMI of this person to drop to 20. Take the metabolizable energy content of 1 kg of body fat to be 33,100 kJ.

Answer 1

The time taken for the BMI of the person to drop to 20 is 2606.46 years

Step-by-step explanation:

Here we have, BMI₁ = 30

BMI₂ = 20

Therefore, since

`BMI = \frac{W (kg)}{H^(2) \hspace {0.06cm}(m^(2) )}`

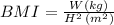
We have 30 = W/1.7² or

W₁ = 1.7²×30 = 86.7 kg

Also, BMI₂ × H² = W₂ = 20 × 1.7² = 57.8kg

Mass to reduced = 86.7 kg - 57.8 kg = 28.9 kg

1 kg of body fat = 33,100 kJ

28.9 kg therefore equals 28.9 × 33100 kJ = 956590 kJ

2 tablespoons of oil = 240 calories = 1004.832 J/day

∴Amount of enerdy excluded per day = 1004.832 J = 1.004832 KJ

Number of days to exclude 956590 kJ = 956590 kJ÷1.004832 KJ/day = 951989.98 days ≈ 2606.46 years.