Question

The function h(t)=16t^+40t models the height in feet of a ball t seconds after it is thrown into the air. What is the maximum height the ball reaches after it is thrown?

Answer 1

1. -4

2. 16

3. 2

4. 16

Explanation:

just did it

Answer 2

The maximum height is 25 feet

Explanation:

The correct question is

The function h(t)=-16t^2+40t models the height in feet of a ball t seconds after it is thrown into the air. What is the maximum height the ball reaches after it is thrown?

we have

`h(t)=-16t^2+40t`

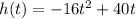
This is a vertical parabola open downward (the leading coefficient is negative)

The vertex represent a maximum

The y-coordinate of the vertex represent the maximum height that the ball reaches

Convert the quadratic equation in vertex form

Factor -16

`h(t)=-16(t^2-(40)/(16)t)`

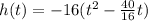
simplify

`h(t)=-16(t^2-(5)/(2)t)`

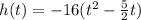
Complete the square

`h(t)=-16(t^2-(5)/(2)t+(25)/(16))+25`

Rewrite as perfect squares

`h(t)=-16(t-(5)/(4))^2+25`

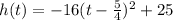
The vertex is the point (1.25,25)

therefore

The maximum height is 25 feet