Question

the weekly mean income of a group of executives is $1,000 and the standard deviation of this group is $100. the distribution is normal. what percent of the executives have an income of $925 or less? group of answer choices

Answer 1

Approximately 23
`\%` percent of the executives have an income of $925 or less

Explanation:

Given -

Mean income
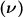`\boldsymbol{(\\u)}` = $1,000

Standard deviation
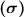`\boldsymbol{(\sigma )}` = $100

Let X be the income of group of executives

what percent of the executives have an income of $925 or less =

`P(X\leq 925 )` =
`P((X - \\u )/(\sigma)\leq (925 - 1000))/(100)`)

=
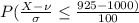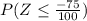`P(Z\leq (-75)/(100) )` Put [
`\boldsymbol{Z = (X - \\u )/(\sigma)}` ]

=
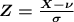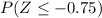`P(Z\leq -0.75)` Using Z table

= .2266

=
`22.66\%`

=
`23%`
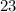
`\%` (Approximately)