The distance between Layana's house and store is 4 units.
Step-by-step explanation:
Given:
Coordinates of Layana's house =

Coordinates of the store =
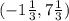
It can also be written as:
Coordinates of Layana's house =

Coordinates of the store =
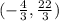
The distance between the house and the shop is:
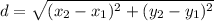
On substituting the value we get:
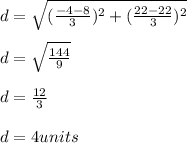
Therefore, the distance between Layana's house and store is 4 units.