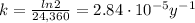Question

The element plutonium-239 is highly radioactive. Nuclear reactors

can produce and also use this element. The heat that plutonium-239 emits has helped to
power equipment on the moon. If the half-life of plutonium-239 is 24,360 years, what is
the value of k for this element?

Answer 1

`2.84\cdot 10^(-5) y^(-1)`

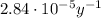
Explanation:

The decay rate of a radioactive isotope (also called activity of the isotope) is given by:

`r=k N`

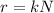
where

r is the decay rate

k is the decay constant

N is the number of nuclei in the radioactive sample

The decay constant of a radioactive isotope is also related to the half-life of the isotope by the formula

`k=(ln2)/(t_(1/2))`

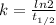
where

`t_(1/2)` is the half-life of the isotope, which is the time taken for the sample to halve, compared to its initial amount

In this problem, the half-life of plutioniun-239 is

`t_(1/2)=24,360 y`

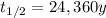
Therefore, the k-factor (decay constant) is:

`k=(ln 2)/(24,360)=2.84\cdot 10^(-5) y^(-1)`