Answer:
Explanation:
The answer given below is not in the form you need. You need the form that includes radicals, not decimals. The formula for the half angle of sin is
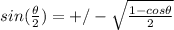 so we need to find out what theta is. If our problem is
so we need to find out what theta is. If our problem is
 , to get that into half angle form, it would be rewritten as
, to get that into half angle form, it would be rewritten as
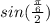 so
so
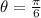
Look to your unit circle to find the EXACT VALUE of the cos of that angle.

Filling that into the formula for the half angle sin:
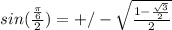 Doing a bit of simplifying gives you
Doing a bit of simplifying gives you
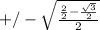 and
and
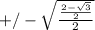 and
and
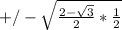 gives you
gives you
 which finally simplifies to
which finally simplifies to
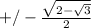
That's the answer in exact format.