Question

9.

The distance (in kilometers) Dora hiked is modeled as a function of time. Consider this graph of the
function. Find the average rate of change in distance hiked, measured in kilometers per hour, between 8:30 a.m to 1:30 p.m. Enter your answer to the nearest whole number.

Answer 1

Given:

The distance (in kilometers) Dora hiked is modeled as a function of time.

We need to determine the average rate of change in distance hiked, measured in kilometers per hour, between 8:30 am to 1:30 pm.

Average rate of change:

Let us write the time and the distance hiked in coordinates for the time 8:30 am and 1:30 pm.

Thus, the coordinates are (8.30, 4) and (1,12)

The average rate of change can be determined using the formula,

`m=(y_2-y_1)/(x_2-x_1)`

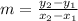
Substituting the points (8.30, 4) and (1.30,12), we get;

`m=(12-4)/(1.30-8.30)`

`m=(8)/(-7)`

`m=-1.14`

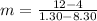
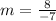
Rounding off to the nearest whole number, we get;

`m=-1`

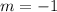
Therefore, the average rate of change is -1.