Answer:
1. The air speed at which wind tunnel should be ran in order to achieve similarity = 61.423 m/s
2. The drag force on the prototype submarine at the conditions given above = 10.325 N.
Step-by-step explanation:
1. Here we have
Density of water at 15 °C = 999.1 kg/m³
Density of air at 25 °C = 1.184 kg/m³
 at 15 °C = 1.138 × 10⁻³ kg/(m·s)
at 15 °C = 1.138 × 10⁻³ kg/(m·s)
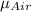 at 25 °C = 1.849 × 10⁻⁵ kg/(m·s)
at 25 °C = 1.849 × 10⁻⁵ kg/(m·s)
The formula is

Where:
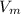 = Velocity of the model =
= Velocity of the model =
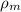 = Density of the model medium at the medium temperature = 1.184 kg/m³
= Density of the model medium at the medium temperature = 1.184 kg/m³
 = Length of the model = 1/8 ×
= Length of the model = 1/8 ×
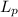 = 0.28 m
= 0.28 m
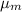 = Dynamic viscosity of model medium at the model medium temperature = 1.849 × 10⁻⁵ kg/(m·s)
= Dynamic viscosity of model medium at the model medium temperature = 1.849 × 10⁻⁵ kg/(m·s)
 = Velocity of the prototype = 0.560 m/s
= Velocity of the prototype = 0.560 m/s
 = Density of the prototype medium at the medium temperature = 999.1 kg/m³
= Density of the prototype medium at the medium temperature = 999.1 kg/m³
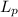 = Length of the prototype = 2.24 m
= Length of the prototype = 2.24 m
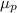 = Dynamic viscosity of prototype medium at the prototype medium temperature 1.138 × 10⁻³ kg/(m·s)
= Dynamic viscosity of prototype medium at the prototype medium temperature 1.138 × 10⁻³ kg/(m·s)
Therefore
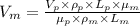
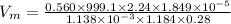 = 61.423 m/s
= 61.423 m/s
The air speed at which wind tunnel should be ran in order to achieve similarity = 61.423 m/s
2. The drag force on the prototype is given by
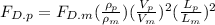
Where:
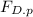 = Drag force of the prototype
= Drag force of the prototype
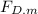 = Drag force of the model
= Drag force of the model
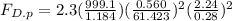 = 10.325 N
= 10.325 N
The drag force on the prototype submarine at the conditions given above = 10.325 N.