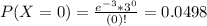Question

Inquiries arrive at a record message device according to a Poisson process of rate 15 inquiries per minute. The probability that it takes more than 12 seconds for the first inquiry to arrive is approximately _________.

Answer 1

0.0498 = 4.98%

Explanation:

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given time interval.

Inquiries arrive at a record message device according to a Poisson process of rate 15 inquiries per minute.

Each minute has 60 seconds.

So a rate of 1 inquire each 4 seconds.

The probability that it takes more than 12 seconds for the first inquiry to arrive is approximately

Mean of 1 inquire each 4 seconds, so for 12 seconds
`\mu = (12)/(4) = 3`

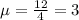
This probability is P(X = 0).

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

`P(X = 0) = (e^(-3)*3^(0))/((0)!) = 0.0498`