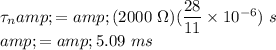Question

A series RC circuit, which is made from a battery, a switch, a resistor, and a 4-μF capacitor, has a time constant of 8 ms. If an additional 7-μF is added in series to the 4-μF capacitor, what is the resulting time constant?

Answer 1

The resulting time constant will be
`\bf{5.09~ms}`.

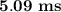Step-by-step explanation:

Given:

the time constant of the RC circuit,
`\tau = 8~ms`

The value of the capacitor in the circuit,
`C_(1) = 4~\mu F`

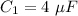
The value of addition capacitor added to the circuit,
`C_(2) = 7~\mu F`

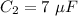
The value of the time constant for a series RC circuit is give by

`\tau = RC`

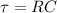
So the value of the resistance in the circuit is

`R &=& (\tau)/(C)\\&=& (8 * 10^(-3)~s)/(4 * 10^-6~F)\\&=& 2000~\Omega`

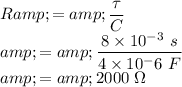
When the capacitor
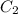`C_(2)` is added to the circuit, the net value of the capacitance in the circuit is

`C &=& (C_(1)C_(2))/(C_(1) + C_(2))\\&=& (4 * 7)/(4 + 7)~\mu F\\&=& (28)/(11)~\mu F`

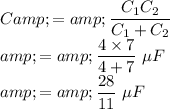
So the new time constant will be

`\tau_(n) &=& (2000~\Omega)((28)/(11) * 10^(-6))~s\\&=& 5.09~ms`