Question

In the United States, 41% of the population have brown eyes. If 14 people are randomly selected, find the probability that at least 12 of them have brown eyes. Is it unusual to randomly select 14 people and find that at least 12 of them have brown eyes? Why or why not?

Answer 1

0.000773 is the probability that atleast 12 out of 14 will have brown eyes.

Explanation:

We are given the following information:

We treat people having brown eyes as a success.

P(people have brown eyes) = 41% = 0.41

Then the number of people follows a binomial distribution, where

`P(X=x) = \binom{n}{x}.p^x.(1-p)^(n-x)`

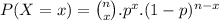
where n is the total number of observations, x is the number of success, p is the probability of success.

Now, we are given n = 14

We have to evaluate:

`P(x \geq 12) = P(x = 12) + P(x = 13) + P(X = 14) \\= \binom{14}{12}(0.41)^(12)(1-0.41)^2 + \binom{14}{13}(0.41)^(13)(1-0.41)^1 + \binom{14}{14}(0.41)^(14)(1-0.41)^0\\= 0.0007 + 0.00007 + 0.000003\\= 0.000773`

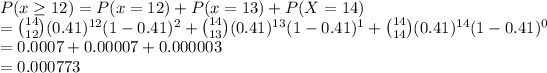
0.000773 is the probability that atleast 12 out of 14 will have brown eyes.

Yes, it is an unusual event due to small probability values.