Question

A survey of 1000 air travelers1 found that prefer a window seat. The sample size is large enough to use the normal distribution, and a bootstrap distribution shows that the standard error is . Use a normal distribution to find a 90% confidence interval for the proportion of air travelers who prefer a window seat. Round your answers to three decimal places.

Answer 1

90% confidence interval for the true proportion of air travelers who prefer the window seat is (0.575, 0.625)

Explanation:

We have the following data:

Sample size = n = 1000

Proportion of travelers who prefer window seat = p = 60%

Standard Error = SE = 0.015

We need to construct a 90% confidence interval for the proportion of travelers who prefer window seat. Therefore, we will use One-sample z test about population proportion for constructing the confidence interval. The formula to calculate the confidence interval is:

`(p-z_{(\alpha)/(2)}\sqrt{(p(1-p))/(n)}, p+z_{(\alpha)/(2)}\sqrt{(p(1-p))/(n)})`

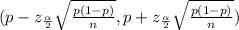
Since, standard error is calculated as:

`SE=\sqrt{(p(1-p))/(n) }`

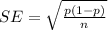
Re-writing the formula of confidence interval:

`(p-z_{(\alpha)/(2)} * SE, p+z_{(\alpha)/(2)} * SE)`

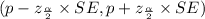
Here,
`z_{(\alpha)/(2)}` is the critical value for 90% confidence interval. From the z-table this value comes out to be 1.645.

Substituting all the values in the formula gives us:

`(0.6 - 1.645 * 0.015, 0.6 + 1.645 * 0.015)\\\\ = (0.575, 0.625)`

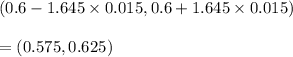
Therefore, the 90% confidence interval for the true proportion of air travelers who prefer the window seat is (0.575, 0.625)