Question

an electric circuit includes a voltage source and two resistances (50 and 75) in parallel. determine the voltage source required to provide 1.6 A of current through the 75 ohm resistance

Answer 1

120 volts

Step-by-step explanation:

Since the two resistances are connected in parallel across the voltage source, the effective resistance of the circuit can be obtained by using the formula.

`(1)/(R_(eq))=(1)/(R_(1))+ (1)/(R_(2))`

given that
`R_(1) and R_(2)` are 50 and 75 ohms respectively, we have the equivalent resistance as:

`(1)/(R_(eq))=(1)/(50)+ (1)/(75)=(1)/(30)`

hence,

`R_(eq)= 30\Omega`

From Ohm's law, voltage = current X resistance.

given that the current through the 75 ohm resistor is 1.6 A

`V= I* R`

`V= 1.6 * 75\Omega`

voltage = 120 Volts.

Because the resistors are connected in parallel, it means that they are connected to the same voltage source.

Hence, the voltage source for the 75 Ohm resistance = 120 volts. This is same for the 50 Ohm resistor.

Answer 2

The voltage source required to provide 1.6 A of current through the 75 ohm resistance is 120 V.

Step-by-step explanation:

Given;

Resistance, R₁ = 50Ω

Resistance, R₂ = 75Ω

Total resistance, R = (R₁R₂)/(R₁ + R₂)

Total resistance, R = (50 x 75)/(125)

Total resistance, R = 30 Ω

According to ohms law, sum of current in a parallel circuit is given as

I = I₁ + I₂

`I = (V)/(R_1) + (V)/(R_2)`

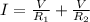
Voltage across each resistor is the same

`1.6 = (V)/(R_2)`

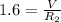
V = 1.6 x R₂

V = 1.6 x 75

V = 120 V

Therefore, the voltage source required to provide 1.6 A of current through the 75 ohm resistance is 120 V.

This voltage is also the same for 50 ohms resistance but the current will be 2.4 A.