Question

47​% of U.S. adults have very little confidence in newspapers. You randomly select 10 U.S. adults. Find the probability that the number of U.S. adults who have very little confidence in newspapers is ​ (a) exactly​ five, (b) at least​ six, and​ (c) less than four

Answer 1

`a. \ P(X=5)=0.2417\\\\b. \ P(X\geq 6)=0.3056\\\\c. \ P(X<3)=0.2255`

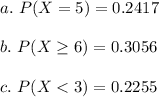
Explanation:

a. This is a binomial probability distribution problem expressed as:

`P(X=x)={n\choose x}p^x(1-p)^(n-x)`

Where:

• 
  `p` is the probability a successful event.
• 
  `x` the number of successful events.
• 
  `n` is the total number of events.

Given n=10, p=0.47 the probability of exactly 5 is calculated as:

`P(X=5)={n\choose x}p^x(1-p)^(n-x)\\\\={10\choose 5}0.47^5(1-0.47)^5\\\\=0.2417`

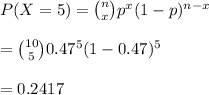
Hence, the probability that exactly 5 adults have very little confidence is 0.2417

b. Given n=10, p=0.47,

-We substitute our values in formula and the probability of at least 6 is calculated as:

`P(X\geq 6)={n\choose x}p^x(1-p)^(n-x)\\\\\\\ \ \ \ \ \ ={10\choose 6}0.47^6(0.53)^4+{10\choose 7}0.47^7(0.53)^3+{10\choose 8}0.47^8(0.53)^2+{10\choose 9}0.47^9(0.53)^1+{10\choose 10}0.47^(10)(0.53)^0\\\\\\=0.1786+0.0905+0.0301+0.0059+0.0005\\\\\\=0.3056`

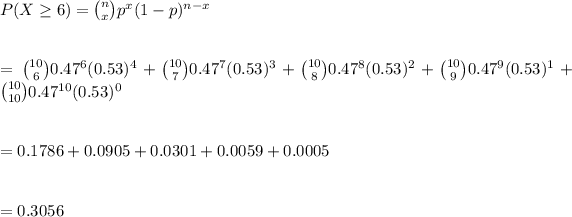
Hence, the probability of at least 6 adults is 0.3056

c. The probability of of less that four adults is calculated as:

`P(X<4)={n\choose x}p^x(1-p)^(n-x)\\\\\\\ \ \ \ \ \ ={10\choose 0}0.47^0(0.53)^(10)+{10\choose 1}0.47^1(0.53)^9+{10\choose 2}0.47^2(0.53)^8+{10\choose 3}0.47^3(0.53)^7\\\\\\=0.0017+0.0155+0.0619+0.1464\\\\\\=0.2255`

Hence, the probability that less that 4 adults have confidence in the newspapers is 0.2255