Question

By what percent will the fraction change if its numerator is

increased by 25% and its denominator is increased by 20%?

Answer 1

The fraction increase by (
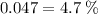`0.047=4.7\:\%` ) if its numerator is

increased by 25% and its denominator is increased by 20%.

Explanation:

• Let 'n' be the numerator

• Let 'd' be the denominator

As

`100\%\mathrm{\:in\:fractions}=\:1`

`25\%\mathrm{\:in\:fractions}=\:(1)/(4)`

`20\%\mathrm{\:in\:fractions}=(1)/(5)`

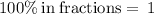
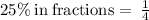
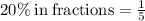
so

Increase in 25% means

`100\%\:+25\%\:=(5)/(4)`

Increase in 20% means

`100\%\:+20\%\:=(6)/(5)`

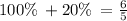
Thus the fraction becomes

`\:((5)/(4)* \:n)/((6)/(5)* \:d)`

`=((5)/(4)n)/((6d)/(5))`

`\mathrm{Divide\:fractions}:\quad ((a)/(b))/((c)/(d))=(a* \:d)/(b* \:c)`

`=(5n* \:5)/(4* \:6d)`

`=(25n)/(24d)`

`=1.0417\left((n)/(d)\right)`

`=1\left((n)/(d)\right)+0.047\left((n)/(d)\right)`

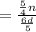
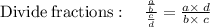
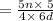
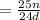
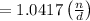
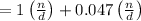
As

`0.047=4.7\:\%`

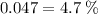
Therefore, the fraction increase by (
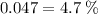`0.047=4.7\:\%` ) if its numerator is

increased by 25% and its denominator is increased by 20%.