Question

What makes this undefined.

Answer 1

Answer: The expression is undefined when x is 3 or -3

Explanation:

The denominator of a fraction can never be 0 because you cannot divide by 0. So all we have to do is determine the value of x when the denominator is equal to 0.

`9-x^(2) =0\\`

We want
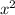`x^2` to be positive so divide everything by -1

`9-x^(2) =0\\\\(9-x^(2))/(-1)=(0)/(-1) \\-9+x^2=0\\x^2-9=0`

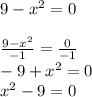
Use difference of two squares (DOTS) to factor

`x^2+9=0\\(x+3)(x-3)=0`

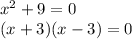
So x cannot be 3 or -3

Answer 2

The expression is undefined when x=3 or -3

Solution:

• An expression is undefined when it's divided by 0.
• In order to make the denominator equal 0, we need to subtract 9-9.
• Notice that x is squared.
• So x times itself should equal 9. What number is it?
• That's right, 3.
• So we have
• 9-(3)^2=9-9=0
• There's also another solution: x=-3 (-3•(-3)=9

Hope it helps.

Do comment if you have any query.