Answer:
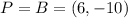
Explanation:
1) Firstly let's place the points in the Cartesian Plane, A is the starting point.
According to the coordinates given:
(Check it out)
2) The distance from A to B, is calculated by:
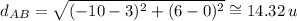
The point P on this line segment AB that is of the distance of 14.32 units is B.
P=B=(6,-10)