Question

A 2.0-cm length of wire centered on the origin carries a 20-A current directed in the positive y direction. Determine the magnetic field at the point x = 5.0 m on the x-axis.

Answer 1

The magnetic field is 1.6 nT in z-direction

Step-by-step explanation:

Given;

Length of wire, L = 2.0-cm

Current in the wire, I = 20-A

point from the axis, r = 5.0 m

Strength of magnetic field can be calculated by applying Biot-Savart equation;

`B = (\mu I)/(2\pi r) ((L)/(√(4r^2 ) +L) )`

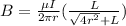
where;

μ is constant given as 4π x 10⁻⁷ T.m/A

Substitute the given values in the equation above and calculate strength of magnetic field.

Since current is moving in y-direction and magnetic field will be caculated from a point 5m in the x-direction, based on vector law, the resultant direction will be z.

`B = (\mu I)/(2\pi r) ((L)/(√(4r^2 ) +L) )\\\\B = (4\pi *10^(-7) *20)/(2\pi *5) ((0.02)/(√(4(5)^2 ) +0.02) )\\\\B = 8*10^(-7) *1.9998 *10^(-3)\\\\B = 1.6*10^(-9) \ T\\\\B = 1.6 \ nT`

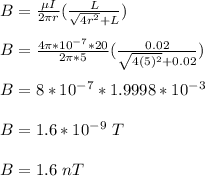
Therefore, the magnetic field is 1.6 nT in z-direction

Answer 2

Answer: field strength = 8x10^-8T

Step-by-step explanation:

At a distance r from a long current carrying conductor of current I,

B = uL/2¶r

Where u = 4¶x10^-7 H/m = permeability of free space.

r = 5m

I = 20A

B = (4¶x10^-7 x 20)/2¶x5

B = 8x10^-8T