Question

You are building identical displays for the school fair using 65 blue boxes and 91 yellow boxes. What is the greatest number of displays you can build using all the boxes?

Answer 1

13

Explanation:

The problem requires us to find the greatest number of displays that can be built using all the boxes.

This is an application of the Greatest Common Factor.

DEFINITION

The GCF of two or more numbers is the biggest number that divides exactly into the numbers.

To find the GCF, we follow the steps below:

Step 1

We break down both numbers into product of prime factors.

65=5 X 13

91 =7 X 13

Step 2

We choose the common factors with the smallest exponent

Therefore the greatest common factor is 13.

The greatest number of displays can be built using all the boxes is 13.

Answer 2

The greatest number of displays that can be built using all the boxes are
`13`

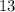
(Using
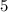`5` blue boxes and
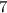`7` yellow boxes for each display).

Explanation:

In order to answer the question, the first step is to divide the number of blue boxes and yellow boxes and look for a common ratio ⇒

`(65)/(91)=(5)/(7)`

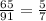
This means that we have a ratio
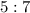`5:7` for blue boxes and yellow boxes.

We find that each display will have 5 blue boxes and 7 yellow boxes.

To find the greatest number of displays that can be built we can do the following calculation

`(65)/(5)=13`

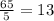
Or

`(91)/(7)=13`

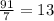
(We can divide the number of blue boxes by its correspond ratio number or the number of yellow boxes by its correspond ratio number)

In each cases the result is 13 displays.

The answer is 13 identical displays