Question

H2(g) + Br2(l) ⇄ 2HBr(g) Kc = 4.8 × 108

Assume initial conditions of 0.400 M H2(g) and excess Br2(l). What is the equilibrium concentration of H2(g)?

Answer 1

• 1.5 × 10⁻⁹M

Step-by-step explanation:

1. Equilibrium equation

• H₂(g) + Br₂(l) ⇄ 2HBr(g)

2. Equilibrium constant

The liquid substances do not appear in the expression of the equilibrium constant.

`k_c=([HBr(g)]^2)/([H_2])=4.8* 10^8M`

3. ICE table.

Write the initial, change, equilibrium table:

Molar concentrations:

H₂(g) + Br₂(l) ⇄ 2HBr(g)

I 0.400 0

C - x +2x

E 0.400 - x 2x

4. Substitute into the expression of the equilibrium constant

`4.8* 10^8=((2x)^2)/(0.400-x)`

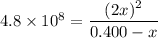
5. Solve the quadratic equation

• 192,000,000 - 480,000,000x = 4x²

• x² + 120,000,000x - 48,000,000 = 0

Use the quadratic formula:

`x=(-120,000,00\pm√((120,000,000)^2-4(1)(-48,000,000))/(2(1))`

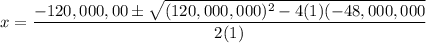
The only valid solution is x = 0.39999999851M

Thus, the final concentration of H₂(g) is 0.400 - 0.39999999851 ≈ 0.00000000149 ≈ 1.5 × 10⁻⁹M